Impedance Spectroscopy for Detecting Phase Transitions in KBr

Team members

Laphas Precmcharoen, Song RunLin, Gao Jia

Introduction

Theory

The theory underlying the experimental study of potassium bromide (KBr) through impedance spectroscopy is based on the principles of solid-state ionics and phase transition physics. Impedance spectroscopy, a powerful technique for characterizing the electrical properties of materials, relies on the measurement of a material's response to an applied alternating current (AC) signal over a range of frequencies. This response provides insights into both the conductive and dielectric properties of the material.

Arrhenius equation

One of the law used in the analysis is the Arrhenius equation, which describes the temperature dependence of reaction rates, and by extension, the conductivity of ionic conductors. The equation is given by:

$\sigma (T)=\sigma _{0}e^{-{\frac {E_{a}}{kT}}}$

where $\sigma (T)$ is the conductivity at temperature $T$, $\sigma _{0}$ is a pre-exponential factor, $E_{a}$ is the activation energy for conductivity, $k$ is the Boltzmann constant, and $T$ is the absolute temperature. The equation decribes the exponential relationship between conductivity and temperature, indicating how the mobility of charge carriers (ions in the case of KBr) increases with temperature.

Materials

1. Impedance Spectroscopy System

2. KBr samples

3. Two-electrode system

4. Frequency generator

Procedure

Palletizing KBr

To prepare the KBr for impedance spectroscopy, the powdered KBr was first palletized. This process involved grinding the KBr into powder to achieve a powdery consistency and then compressing under a pressure of approximately 5 tons using a hydraulic press for approximately 3 minutes, forming a compact, disk-shaped pellet with a diameter of 11.4 mm and a thickness of approximately 2.61 mm. The uniform size and shape of the pellet were crucial for ensuring consistent and reliable impedance measurements.

Grinding KBr into a powdery consistency

compressing powderized KBr under a pressure using a hydraulic press

Application of Silver Paint

After palletization, both sides of the KBr pellet were coated with a thin layer of silver paint. This step was performed carefully to ensure a uniform conductive layer was formed, minimizing any potential impedance contributions from uneven surface conductivity. The silver-coated pellet was then left to dry for 15 minutes, ensuring the paint was fully set and conductive.

Pallets after silver painting

Electrode Setup

Once the silver paint was dry, electrodes were attached to both sides of the pellet. This setup facilitated the application of an alternating current (AC) through the pellet, necessary for impedance measurements. The electrodes were connected to the impedance spectroscopy system.

Impedance Spectroscopy System and Heating

The impedance spectroscopy measurements were conducted using an impedance analyzer capable of capturing impedance data over a broad frequency range. The frequency range for the measurements spanned from 100 Hz to 100,000 Hz, with a total of 60 frequencies measured at evenly spaced logarithmic intervals. This comprehensive frequency range was selected to ensure a detailed characterization of the KBr pellet's impedance properties across different electrical conduction regimes. For the experiment, the KBr pellet, with electrodes attached, was placed in a temperature-controlled chamber within the impedance spectroscopy system. The temperature of the chamber was increased in steps of approximately 10°C, starting from 50°C and reaching up to 250°C. At each temperature step, impedance data were recorded across the predetermined frequency range. This methodical temperature increment allowed for the detailed observation of the KBr pellet's phase transition behavior at each step.

Impedance spectroscopy of KBr across different tempertures

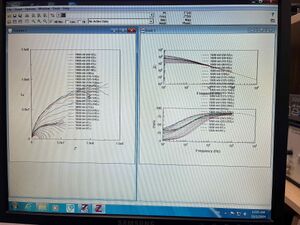
Data Analysis

Impedance data were analyzed and presented as Nyquist plots for each temperature step. The data was fitted using parallel RC circuit. The fitting techniques were employed on these plots to deduce the resistance and capacitance values across the temperature spectrum. From the resistance values, the conductivity was computed using pallet's dimension. The conductivity versus tmeperature data was plotted in the following 2 ways: 1. the conductivity times temperature (σT) versus reciprocal temperature (1/T) graph, 2. the log(σ) versus reciprocal (1/T) temperature graph. These analyses aimed to identify shifts in the slopes of these graphs, which are indicative of phase transitions within the KBr pellet. Special emphasis was placed on changes observed around 190°C, where a noticeable shift in the slopes suggested a phase transition.

The choice to analyze the relationship between (σT) and (1/T) is inspired by the seminal work of Suresh Chandra and John Rolfe, as detailed in their paper "Ionic Conductivity of Potassium Bromide Crystals." Their research demonstrated a clear linear relationship between these variables, highlighting the utility of this approach in predicting conductivity across different temperatures and identifying phase transitions.

In addition to the (σT) versus (1/T) analysis, our study also incorporates the log(σ) versus (1/T) graph, supported by the Arrhenius equation. The Arrhenius law provides a fundamental description of how reaction rates (or, in our case, conductivity) increase with temperature. By plotting the natural logarithm of the conductivity against the reciprocal of the temperature, we can extract the activation energy for the conduction process within the KBr crystals. This relationship is crucial for understanding the temperature dependence of ionic movement and offers additional evidence of phase transitions, especially when anomalies or deviations from the expected linear trend are observed.

Results

The impedance spectroscopy measurements of potassium bromide (KBr) across the temperature range of 50°C to 250°C resulted in a series of Nyquist plots. Each plot displayed a semi-circular arc, the diameter of which varied with temperature, indicating changes in the material's impedance properties. The RC parallel fitting method was employed to approximate the pellet's conductivity and capacitance values at each temperature step. The circle fitting applied to a selected Nyquist plot is illustrated in Figure 1.

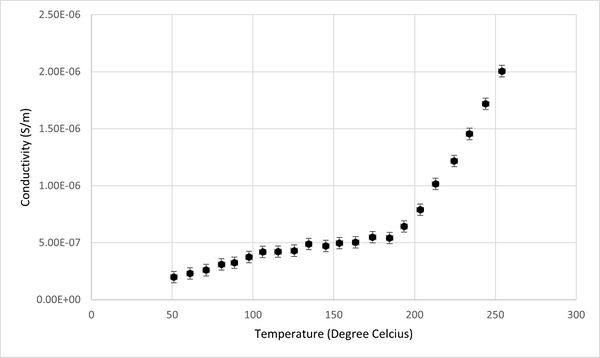
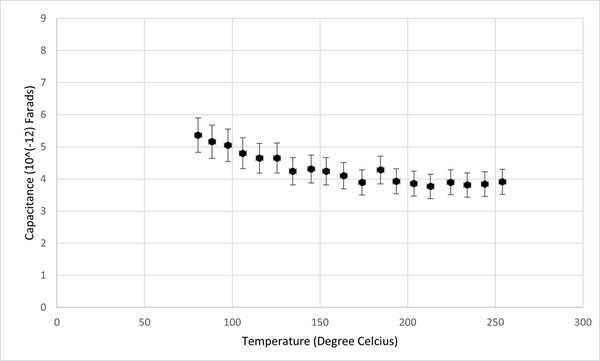
Additionally, the relationship between conductivity, capacitance, and temperature for the KBr pellet was graphically represented, as depicted in Figures 2 and 3. It was observed that the capacitance of KBr remained relatively stable across the temperature range from 50°C to 250°C. This stability suggests that capacitance is not an effective sensing property for identifying phase transitions in KBr. However, a notable variance in the conductivity of the KBr pellet within the same temperature range positions conductivity as a more viable sensor property for the detection of phase transitions. Therefore, the study will concentrate on analyzing conductivity as the primary sensor property.

Figure 2:The graph between approximated conductivity obtained from circle fitting versus the temperature of the palettized KBr

Figure 3:The graph between approximated capacitance obtained from circle fitting versus the temperature of the palettized KBr

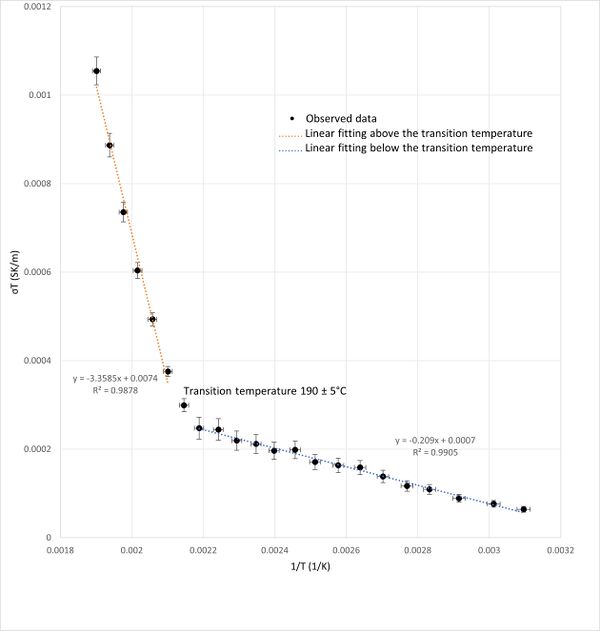
A graph plotting (σT) versus (1/T) was presented in Figure 4.The graph revealed a linear relationship in two distinct segments, separated by a noticeable slope change (inflection point). Therefore, a linear fit of these two segments is used (Figure 4). This inflection point, occurred around 190°C, indicates a phase transition temperature for KBr. The linearity of the segments before and after the transition point agrees with (reference) as explained in the Discussion section.

Figure 4:The graph between conductivity times temperature against reciprocal temperature showing a linear relationship in two distinct segments indicating a phase transition at around 190°C

Similarly, the plot of the logarithm of conductivity (log(σ)) against reciprocal temperature (1/T) shown in figure 5 exhibited two linear regions intersecting at the same transition temperature of approximately 190°C. The two straight regions are consistant with the Arrhenius law. The change in the slope at approximately 190C indicates a shift in activation energy at for ionic conductivity. This shift in activation energy implies a structural phase transition within the KBr crystal lattice, as it indicates changes in the energy barrier of ionic movement, reflecting an alteration in the crystal's structural configuration.

Figure 4:The graph between log of conductivity against reciprocal temperature also showing a linear relationship in two distinct segments indicating a phase transition at around 190°C

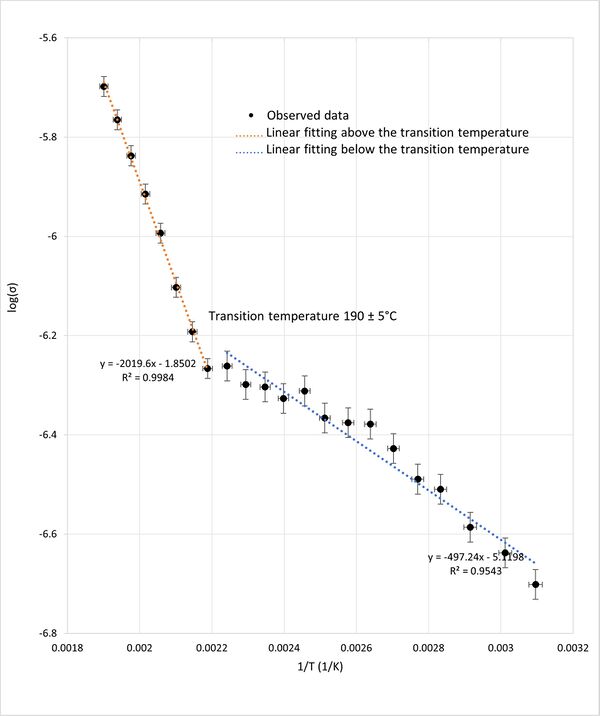
The identification of a transition temperature around 190°C, consistent across both graphical analyses, highlights the sensitivity of impedance spectroscopy in detecting phase transitions in KBr. This temperature mark serves as a critical point where the material's conductive properties undergo significant changes, likely due to alterations in the crystal structure which will be discussed in more detailed in the discussion section.

Discussion

The data suggests that semiconductor KBr undergoes a phase transition around 190°C. Before and after this temperature, the sample points approximately follow linear fitting, as evidenced by both the conductivity times temperature against reciprocal temperature and the logarithm of conductivity against reciprocal temperature graphs. Comparing this observation with previous studies on phase transitions in two other semiconductors, NaNbO3 and BaZrxTi1-xO3, we can infer that in KBr potential structural changes are occuring around 190°C, indicative of phase transition.

In the case of NaNbO3, research indicates that a phase transition occurs from pseudocubic to cubic symmetry in single crystal NaNbO3 at 640°C. Additionally, the permittivity curve shows three local maxima at 250°C, 448°C, and 574°C, corresponding to orthorhombic to tetragonal, tetragonal to pseudocubic’, and pseudocubic” to another phase transition respectively.

For BaZrxTi1-xO3, the study first established different compositions (e.g., BZT1, BZT2, BZT3, etc.) and measured transitions from 20°C to 225°C. The results indicated a sharp transition at the phase transition temperature (Tm) for BZT1, with another transition at a lower temperature (Td, orthogonal to tetragonal). Other compositions exhibited only one sharp transition at Tm, with Tm decreasing gradually with increasing x.

These studies elucidate structural changes during phase transition points, which could explain the observed slope changes in our graph. This further suggests that our experiment detects the phase transition in KBr by sensing conductivity as a function of temperature.

Conclusion

References

Timeline

Timeline

5/3	Learn how to do the Impedance spectrocopy and how to palletize samples
7/3	Palletize powdered KBr
12/3	Patelletize KBr into different thicknesses
14/3	Silver paint both sides of the pallets
19/3	1st attempt on the impedance spectroscopy across different temperatures
20/3	final attempt on the impedance spectroscopy across different temperature from 50°C - 255°C of the pallet with diameter 11.4 mm and thickness 2.61 mm
21/3	Do the impedance spectroscopy while applying bias DC voltage from 0V to 5V of the pallet with diameter 11.7 mm and thickness 3.54 mm and do the impedance spectroscopy on the pallets with different thicknesses
26/3	Fit the nyquist plot of the temperature data with a semi circle to get approximated resistance and capacitance
2/4	Discuss the result and fit the DC dependent data with semi circle
4/4	Analyze the data and discuss
9/4	Discuss the possible mechanism of phase transition and updating wiki page